10.2 Lineare Differentialgleichung zweiter Ordnung mit konstanten Koeffizienten

, , sei gegebene Funktion, die stetig ist.

• , ist ein komplexer Vektorraum.
• Es sei . Dann gilt:

Man erhält alle Lösungen von , indem man zu einer Lösung alle Lösungen aus addiert.

Beweis:

Lösung:

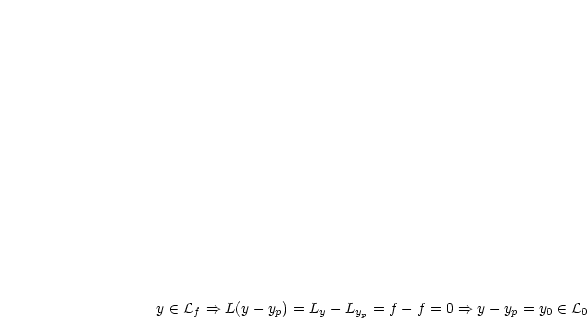
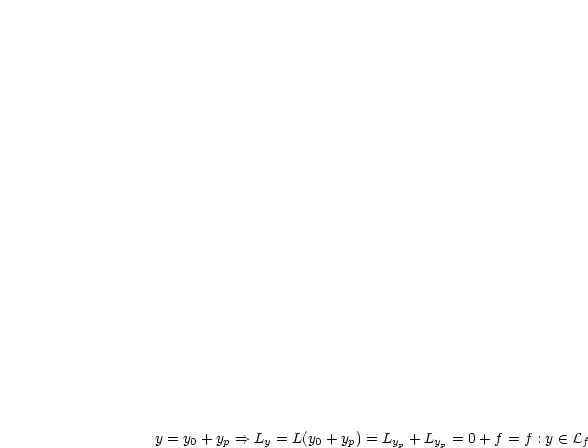
Wir verwenden den Ansatz . sei somit eine Lösung dieser Differentialgleichung. Wenn wir diesen Ansatz in die Differentialgleichung einsetzen und umformen, erhalten wir:

Mit erhält man:

• Ist Lösung von (2), so ist Lösung von (1).
• Ist Lösung von (1), so ist Lösung von (2).

Ab jetzt:

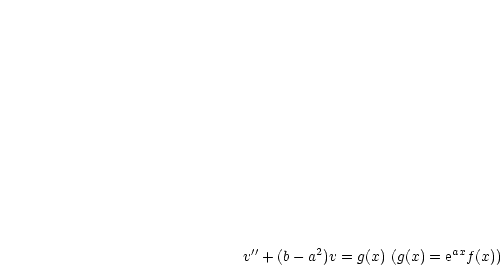
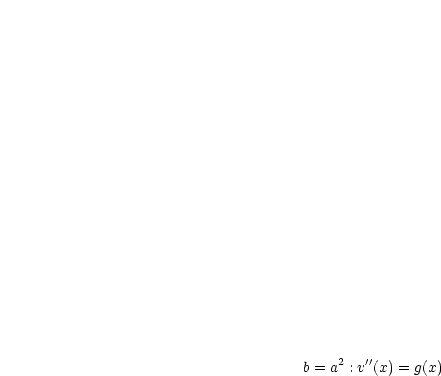
Jetzt müssen wir nur noch zweimal integrieren:

Wir integrieren partiell:

Übung:

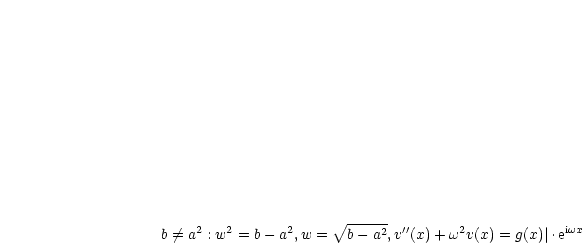
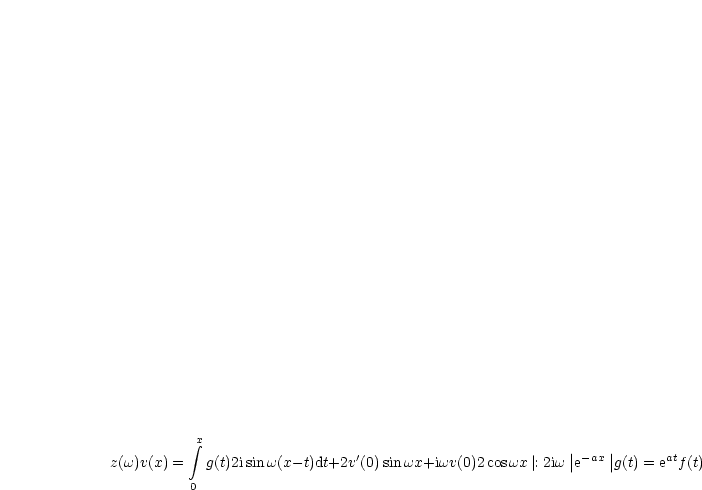
Wir subtrahieren die beiden Gleichungen voneinander:

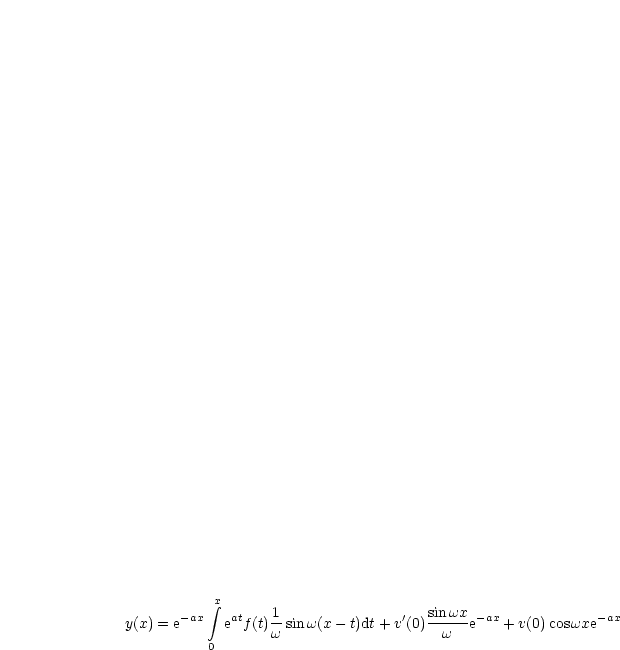
Allgemeine Lösung , ist beliebig, aber konstant.

• , :

• , ,

  (10.2)

  Andere Strategie zur Lösung:

  • Homogene Gleichung, Ansatz:

  • Die inhomogene Lösung erhält man durch Variation der Konstanten, also durch folgenden Ansatz:

    , sind nun zu berechnen.

    

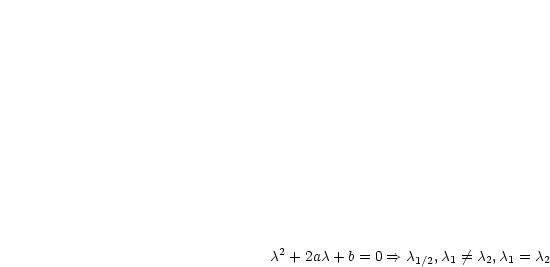
    Eine alternative Variante, die stets funktioniert, ist folgende:

    • Berechne
    • 
    

  Zusammenfassung:

  

  Dies ist eine lineare Differentialgleichung -ter Ordnung mit konstanten Koeffizienten.

  

  Gilt , so spricht man von homogenen Differentialgleichungen, sonst von inhomogen.

  Weiteren Typen von Differentialgleichungen:

  

  Lösung mit speziellem Ansatz, um Problem auf bekannte Differentialgleichungen zurückzuführen.

  
  • EULERsche Differentialgleichung, linear, -te Ordnung, Koeffizienten nicht konstant
  • Lösung erfolgt mit speziellem Ansatz.

  Sind und zwei Lösungen einer linearen Differentialgleichung, so löst auch die Differentialgleichung.

  Beispiel:

  ist eine nichtlineare inhomogene Differentialgleichung 1.Ordnung. Wir verwenden zur Lösung den Ansatz:

  
  

  Durch Einsetzen in die Differentialgleichung folgt:

  
  
  
  
  
  
  
  
  
  

  Lösung der ursprünglichen Differentialgleichung:

  

  Beachte:

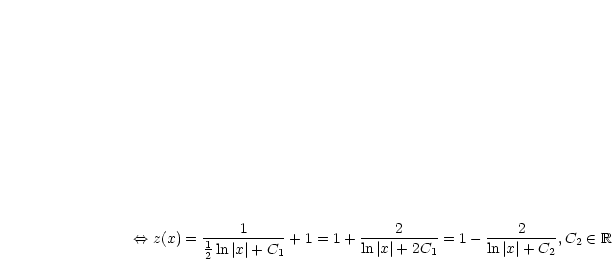
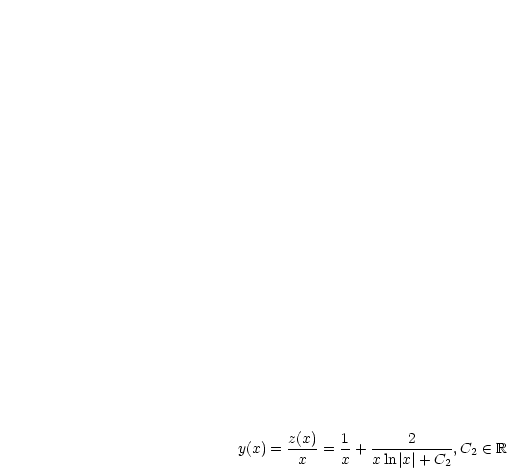
  bzw. löst Differentialgleichung ebenfalls. Dies ist in unserer Lösung für erhalten.

  Beispiel:

  Man löse folgende Differentialgleichung:

  

  Wir machen folgende Substitution:

  

  Damit gilt:

  

  Durch Einsetzen folgt:

  
  

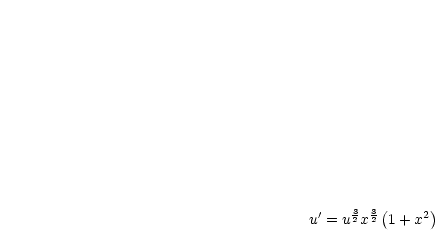
  Durch Integration gilt dann:

  
  
  

  Also erhalten wir für :

  

  Beispiel:

  

  Dies ist eine EULERsche Differentialgleichung.

  Lösungsansätze:

  • Möglichkeit 1:
    

  • Möglichkeit 2:

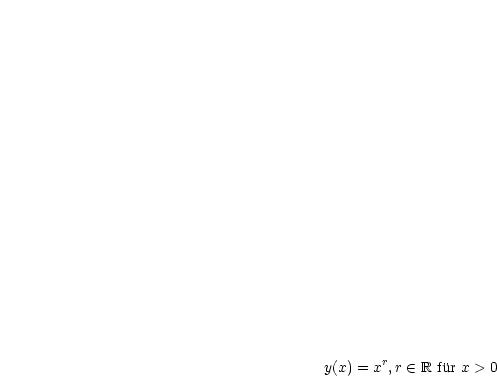
    Wir verwenden folgende Substitution:

    
    

    Damit folgt:

    
    

  Einsetzen in die Differentialgleichung liefert:

  

  Homogene Differentialgleichung:

  

  Man verwendet hier den Ansatz , .

  

  Damit erhalten wir das Charakteristische Polynom:

  
  

  Alle Lösungen der homogenen Differentialgleichung:

  

  Inhomogene Differentialgleichung:

  Betrachten wir nun die Differentialgleichung . Deren Störfunktion ist ; dies ist ein Polynom 1.Grades. Außerdem liegt keine Resonanz vor. Damit verwenden wir als Ansatz zur Lösung des inhomogenen Problems 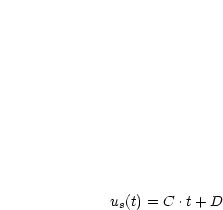, also ebenfalls ein Polynom 1.Grades (). Durch Einsetzen folgt:

  
  

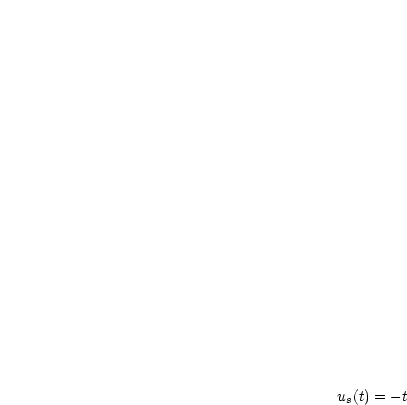
  Die Differentialgleichung ist linear; die Gesamtlösung ergibt sich durch Superposition der Lösung der homogenen und der inhomogenen Gleichung:

  

  Bemerkungen:

  
  

  Rücksubstitution:

  

  [prev] [prev-tail] [front] [up]